Combustion, Explosion, and Shock Waves, Vol. 38, No. 5, pp. 552–558, 2002

Parametric Analysis of the Ignition Conditions of

Composite Polymeric Materials in Gas Flows

UDC 536.46

G. N. Isakov

1

Translated from Fizika Goreniya i Vzryva, Vol. 38, No. 5, pp. 70–77, September–October, 2002.
Original article submitted June 8, 2001; revision submitted November 5, 2001.

This paper reports an experimental procedure and results of studying the ignition
of variously shaped specimens of composite polymeric materials in gas flows. The
main ignition mechanism under these conditions is the propagation of the heteroge-
neous oxidation of soot-formation products (carbon black and pyrocarbon) from the
surface of contact with the oxidizer into the interstitial space controlled by diffusion.
A balance relation between heat input and heat removal on the porous reacting sur-
face is obtained to estimate the ignition conditions of composites under the conditions
studied.

Key words: ignition, composite polymeric material, heating, cooling, porosity,

heterogeneity, pyrocarbon, gas flows.

INTRODUCTION

At present, composites based on polymeric binders

with reinforcing carbon fibers and/or glass fabric [1]
have been widely used to manufacture pipelines, chim-
ney and ventilation gas flues, reservoirs and tanks for
transportation of corrosive media, etc. [1–3]. This re-
duces considerably the cost of installation and opera-
tion of process equipment in the oil, chemical, and gas
industries. The fiberglass and coal plastics used in load-
bearing members must be tested for fire safety in acci-
dental situations with pulsed thermal action from dif-
ferent sources, for example, for accidents with forma-
tion of “fire balls” [2], for calculation of the damaging
fire factors under depressurization of railway tanks with
liquefied hydrocarbon gases [3], etc.

The processes observed under these conditions can

be imagined as follows: the surface of a specimen of a
composite polymeric material (CPM) is first heated for
a short time by a high-intensity heat source (convective
[4, 5], radiative [2, 3], or radiative–convective [5]) and is
then subjected to a flow of a cold gaseous oxidizer. In
the heated layers of CPM, this can cause thermal de-
struction of the polymeric binder [4, 5] with formation of
a porous coked layer and deposition of carbon black and

1

Institute of Applied Mathematics and Mechanics,
Tomsk State University, Tomsk 634050;
[e-mail].

pyrocarbon on the pore surface and walls [4]. When in
contact with the gaseous oxidizer, they react chemically
to release a considerable amount of heat, which leads
to self-heating of the surface, ignition, and subsequent
combustion [4]. In addition, the gaseous products of
thermal destruction can enter a chemical reaction with
oxygen in the boundary layer [4, 5].

Under such complex conditions, the available fire

resistance standards [6, 7] for structures made of CPM
are insufficient because they ignore mechanisms of heat
and mass transfer and ignition. As is correctly pointed
out in [8], it is not always possible to establish an em-
pirical relationship between the oxygen index [6–8] and
the carbonized porous layer formed upon the thermal
destruction of CPM. In addition, restrictions on the
magnitude of critical heat flows and the time of their
action on CPM [3] leading to ignition are not satisfied.

The goal of the work described here was to develop

a procedure for estimating ignition conditions for CPM
using balance relations between generalized (dimension-
less) parameters of heat and mass transfer on porous
specimens of various configurations from data of mea-
surements of temperature profiles in gas and condensed
phases.

552

0010-5082/02/3805-0552 $27.00 c

2002

Plenum Publishing Corporation

Parametric Analysis of the Ignition Conditions for Composite Polymeric Materials

553

Fig. 1. Diagram of the thermal experiment with heat-
ing of a plate of CPM in a high-temperature gas flow (a)
and cooling in a flow of a cold oxidizer (b): 1) specimen;
2) holder; 3) gas generator; 4) boundary layer; 5) chan-
nel; 6) probe for measuring boundary-layer temperature;
7) microthermocouples; 8) probe for temperature mea-
surements in the solid phase.

EXPERIMENTAL PROCEDURE

The specimens studied had a hemispherical shape

[5], the shape of plates [4, 5], and a tubular shape with
relative length L/d

≥ 10 and relative wall thickness

h/d

≤ 10 (L, h, and d are the length and thickness of

the walls and the diameter of the cylinder, respectively).
The following types of CPM were studied: PT-10 texto-
lite [5]; fiberglass plastics based on IF-ED6 epoxyphe-
nol resin [1, 4] and two types of glass fabric, namely,
TSU 8/3 [1] (symbolic notation CPM-1) and VPR-10
[1] (symbolic notation CPM-2); fiberglass plastic based
on EKhD resin with TSU8/3 glass fabric [1] (symbolic
notation CPM-3).

The ignitability of the fiberglass plastics was stud-

ied by the following procedure (Fig. 1). Tested spec-
imens in the shape of a hemisphere or a plate heat-
insulated from the butt ends was set in a holder and
heated for time t

heat

in a flow of a high-temperature gas

from a gas generator. The plate is aligned with the axis
of the generator and the forepart of the holder shaped
as an acute-angled leak, as shown in Fig. 1a. After that,
the specimen is automatically placed in a cold oxidizer
flow (see Fig. 1b) with known velocity U

ox

, temperature

T

ox

, and oxygen concentration C

ox

. After preheating for

Fig. 2. Experimental curves of T

s

(t) for hemispher-

ical specimens of different CPM: curve 1 refers to
CPM-2 for C

ox

= 0.98 and β

x

= 80 sec

−1

, curve 2

refers to PT-10 for C

ox

= 0.5 and β

x

= 130 sec

−1

,

curve 3 refers to CPM-2 for C

ox

= 0.98 and β

x

=

115 sec

−1

, and curve 4 refers to PT-10 for C

ox

= 0.98

and β

x

= 80 sec

−1

.

a specified time t

heat

using an electrostop watch and a

special device, the specimen is automatically introduced
into the unit shown schematically in Fig. 1b.

Space–time profiles for the gas and condensed

phases on plate specimens [4] were measured by mi-
crothermocouple probes. The probe for measuring tem-
perature profiles in the boundary layer above the spec-
imen surface at t > t

heat

consisted of 3 or 4 Chromel–

Alumel microthermocouples [5], whose outputs were
registered in time by a recorder. The probe for mea-
suring solid-phase temperature also consists of 4 mi-
crothermocouples embedded at different distances from
the surface during the manufacture of the specimen.

Figure 2 shows oscillograms of the surface temper-

ature T

s

for hemispherical specimens. The specimens

were heated in a burner flame [4, 5] for time t

heat

and

then were placed (time of placement

≈0.04 sec) [4, 5]

in a gaseous oxidizer flow at T

ox

= 295 K and differ-

ent values of C

ox

and β

x

, where β

x

= 1.5U

ox

/r

s

is a

gas-dynamic parameter [5] and r

s

is the radius of the

hemisphere.

Temperature measurements in the gas and con-

densed phases of CPM-2 plate specimens performed by
rolled U-shaped Chromel–Alumel microthermocouples
50 µm thick (measurement error

≈8% [4, 5]) showed the

presence of temperature “humps” in the coked layer [4].

554

Isakov

Fig. 3. Curves of T

s

(t) (1) and ∆T (t) (2) for plate

specimens of CPM-2 at t > 4 sec (T

ox

= 295 K,

C

ox

= 0.98, U

ox

= 0.5 m/sec, and t

heat

= 7.6 sec).

In this case, the variation of the temperature difference
∆T (t) in the gas phase [T

g

(t)] and on the surface [T

s

(t)]

is equidistant to the temperature T

s

(t), which suggests

that the gas-phase processes are quasistationary [4, 5]
at t > 4 sec (Fig. 3). Disturbance of the equidistant na-
ture at t < 4 sec is apparently explained by additional
heat input due to burnup of soot deposits on the mi-
crothermocouple surface during probe measurements in
the gas phase.

Heat tests of tubular CPM specimen were per-

formed on the experimental facility shown schematically
in Fig. 4a. The facility consists of a cylindrical steel
casing with a tubular CPM specimen placed in it. The
butts of the specimen are tightly fitted to the flanges of
the casing. The upper butt of the casing is shaped as
a confusor, so that the high-temperature gas jet issu-
ing from the gas-generator nozzle has a steady velocity
profile at the entrance to the specimen during the en-
tire operation of the gas generator. The presence of a
convergent part of the casing eliminates the effect of
the initial segment on the formation of the boundary
layer in a hollow specimen of CPM. At the bottom of
the casing there is a stable basis with a coaxial outlet.
At t > t

heat

, the casing with the specimen is displaced

and aligned with the pipe and the hole through which a
cold gaseous oxidizer flows see Fig. 4b). A grid is used
to produce a uniform velocity profile at the entrance to
the cavity of the specimen.

Distributions of the surface temperature T

s

along

the x coordinate were measured in time by four rolled
thermocouples, denoted in Fig. 4 by TC-1–TC-4. Tem-
perature profiles along the y coordinate in any section
of tubular specimens were determined by microthermo-

Fig. 4. Diagram of tests of tubular CPM specimens of
CPM: gas-generator nozzle (1), high-temperature jet (2),
cylindrical steel casing (3), specimen (4), basis (5) with
an outlet (6), fitting pipe (7) with a hole (8) through
which a cold gaseous oxidizer flows, grid (9), thermocou-
ples (10), and flame (11).

couple probes similar to those shown in Fig. 1. Ignition
of the specimens, visualization of the flame above the
upper butt of the casing, and the dynamics of flame
propagation were recorded by photoelectric cells and a
movie picture camera. Figure 5 shows oscillograms of
the surface temperature in four sections along a tube of
CPM-2 (along the x coordinate) obtained by Chromel–
Alumel microthermocouples [4, 5]. It is evident that the
surface temperature variation for tubular specimens is
similar in nature to that for hemispherical (see Fig. 2)
and plate (see Fig. 3) specimens of different CPM. This
suggests the existence of a unified ignition mechanism
for CPM under the given heat- and mass-transfer con-
ditions.

ANALYSIS OF THE
IGNITION MECHANISM

To understand the mechanism of ignition of CPM

under the conditions described above, it is necessary to
know the time dependences of mass loss for the speci-
mens ∆M (t). To this end, it is necessary to experimen-
tally determine the rate of mass ablation (ρv)

s

using

Parametric Analysis of the Ignition Conditions for Composite Polymeric Materials

555

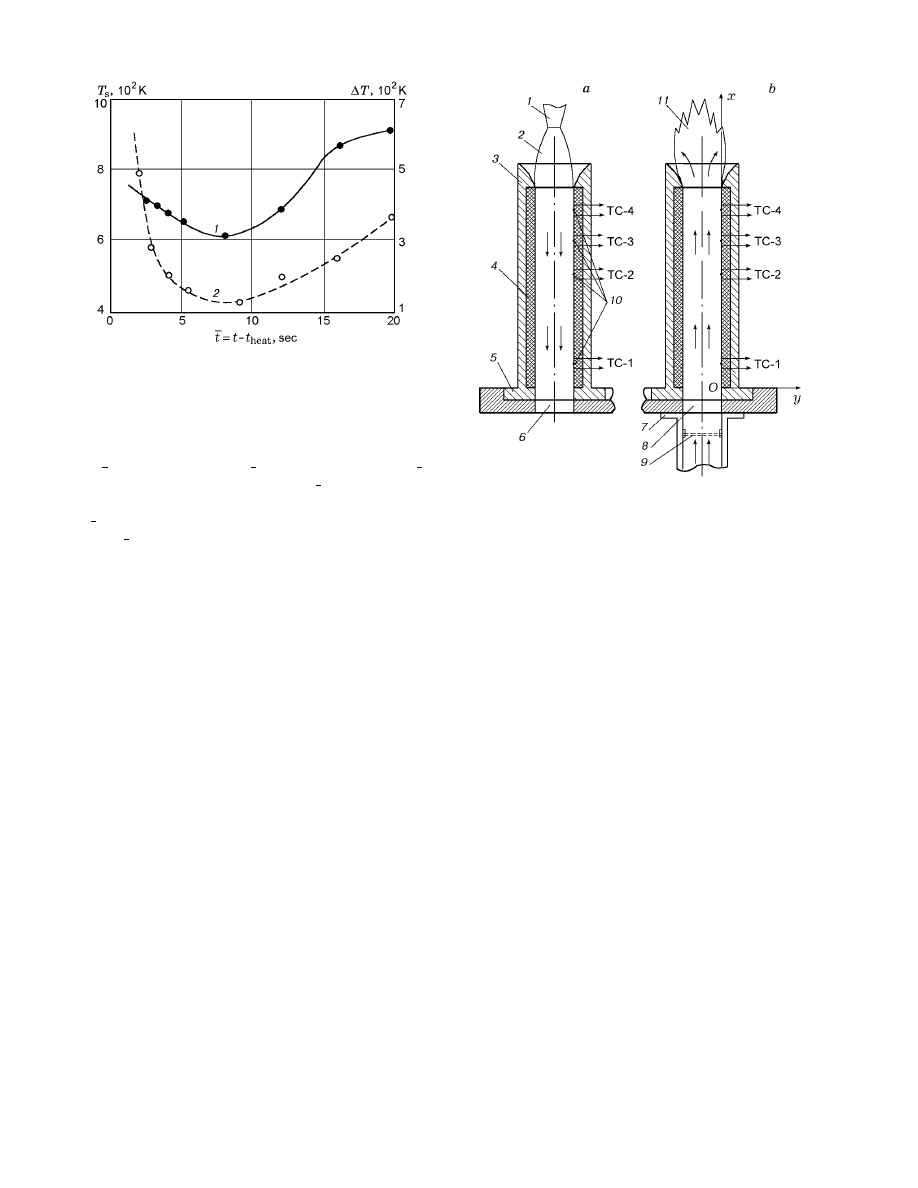
Fig. 5. Curves of T

s

(t) for a tubular specimen of

CPM-2 for various values of the longitudinal coordi-
nate: x = 2.5

·10

−2

(1), 12.5

·10

−2

(2), 17.5

·10

−2

(3),

and 22.5

· 10

−2

m (4); solid curves refer to ignition

and dashed curves refer to extinction.

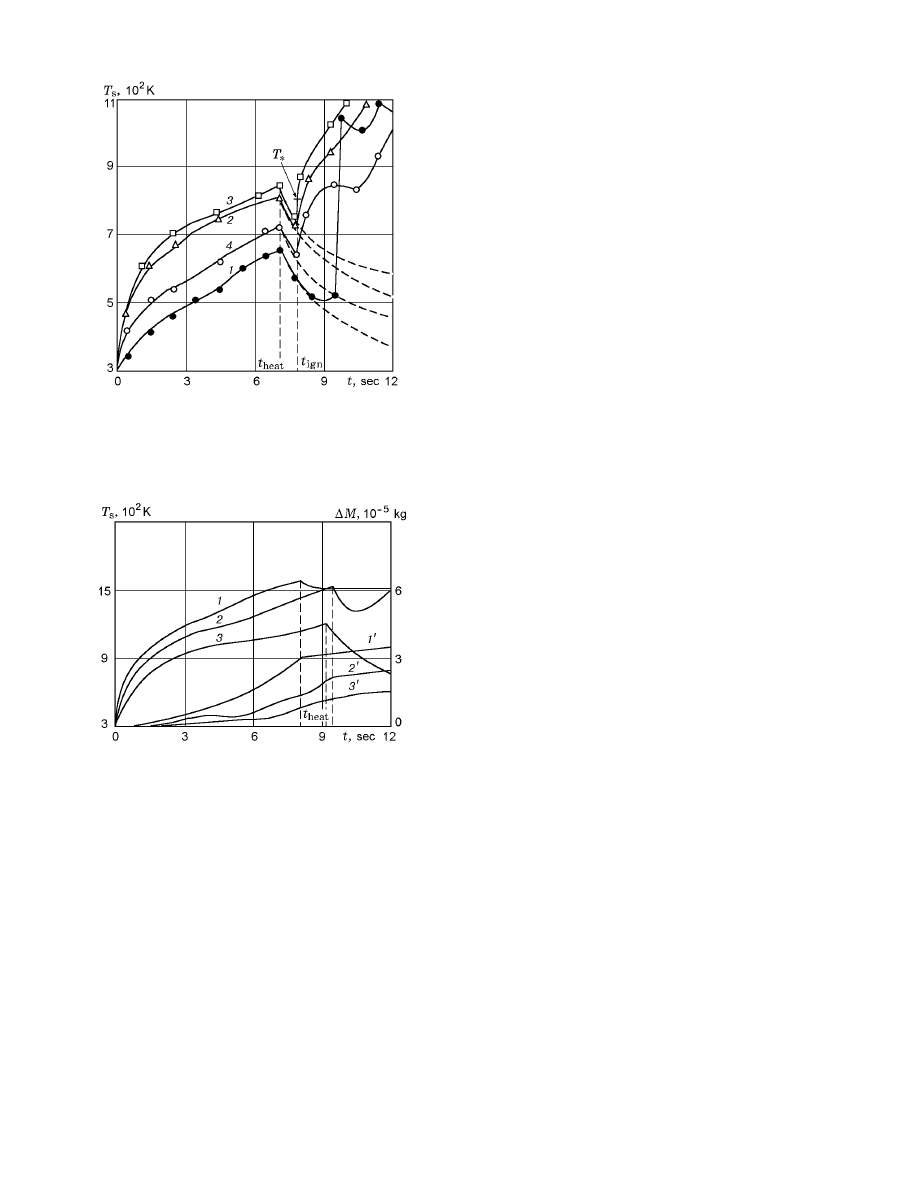
Fig. 6. Experimental curves of surface temperature
(curves 1–3) and loss of mass (curves 1

0

–3

0

) for CPM-

3 fiberglass plastic.

the procedure described in [5, 9, 10]. In such experi-
ments, at the end of heating for time t

heat

, the high-

temperature flame is cut off by a damper and the chan-
nel is vented by a gaseous oxidizer flow with specified
values of T

ox

, U

ox

, and C

ox

. Figure 6 shows experi-

mental curves of T

s

(t) and ∆M (t) for CPM-3 fiberglass

plastic given in [10].

An analysis of results of the experiments (see

Figs. 2, 3, 5, and 6) shows that three regimes of un-
steady heat- and mass-transfer processes are possible
under these conditions:

1) An ignition regime, in which the surface tem-

perature at t > t

heat

first decreases and then gradu-

ally increases with time and reaches a steady-state value
(specimen burns); this regime is observed for specimens
of all types (see curves 1–4 in Fig. 2 for hemispherical
specimens, curves 1–4 in Fig. 5 for tubular specimens,
curves 2 and 2

0

in Fig. 6 for plate specimens [10]);

2) For high values of T

s

at t > t

heat

, the surface

temperature decreases only slightly (see curves 1 and 1

0

in Fig. 6) and enters a steady combustion regime without
formation of a minimum on the curve of T

s

(t);

3) An extinction regime, in which ignition condi-

tions are not attained and at t > t

heat

there is a mono-

tonic increase in temperature with time (see the dashed
lines in Fig. 5 and curves 3 and 3

0

in Fig. 6).

The existence of the above-mentioned regime indi-

cates that under the conditions studied, there are crit-
ical conditions for ignition of CPM. For quantitative
assessment of the critical conditions, Table 1 gives test
data on the ignitability of two types of fiberglass plastics
after preheating taken from [10].

The dynamics of surface temperature for variously

shaped specimens of different CPM (see Figs. 2, 3, 5,
and 6) and the existence of three regimes (see Fig. 6)
and critical conditions (see Table 1) suggest that the
ignition of CPM in gas flows with variable properties
proceeds by the following mechanism (proposed for the
first time in [4]).

At t = t

heat

, when the specimen

is placed in a cold oxidizer flow, heterogeneous oxida-
tion of the soot film begins on the wall. However, the
heat of this reaction is insufficient.

As a result, the

surface temperature decreases due to cooling and the
rate of mass ablation (ρv)

s

tends to zero. After reach-

ing a certain minimum value T

∗

, the surface tempera-

ture begins to increase, which is explained by burnup
of the carbon black film on the surface and opening of
the pores. Thus, oxygen from the incident flow diffuses
into the depth of the porous layer and enters a chemi-
cal reaction with the product of soot formation (carbon
black and pyrocarbon) [4]. The heat release due to this
exothermic heterogeneous reaction on the highly devel-
oped interstitial surface becomes sufficiently high that
self-heating of the a porous layer and surface begins.
This is also favored by the heat release due to the gas-
phase reactions of oxidation of fine sooty inclusions, as
well as of hydrogen, methane, and carbon oxide [10–12]:
2H

2

+ O

2

=2H

2

O, CH

4

+ 2O

2

= CO

2

+ 2H

2

O, and

2CO+ O

2

= 2CO

2

.

The experiments (see Fig. 3) confirm the quasis-

tationarity of the gas-phase processes.

Therefore, to

determine the integral heat effect on the surface due to
gas-phase reactions in the flame, we use the expressions
for the effective heights of individual flames (Y

f

) and

556

Isakov

TABLE 1

Test Results on the Ignitability of Two Types of Fiberglass Plastics

Material

T

heat

, K

T

ox

, K

C

ox

U

ox

, m/sec

Result

CPM-1

1600

1070

0.23

4.6

Ignition

CPM-1

1600

800

0.23

6.7

No Ignition

CPM-1

1830

292

0.23

4.3

—

00—

CPM-1

1630

292

0.98

0.8

Ignition

CPM-3

1360

1128

0.23

9.2

No ignition

CPM-3

1535

1128

0.23

9.2

Ignition

CPM-3

1270

292

0.98

0.9

No ignition

CPM-3

1210

292

0.98

1.9

Ignition

the mass combustion rates (m

i

) of fine soot inclusions

(H

2

, CH

4

and CO), adopting the model of competitive

flames [5]:

Y

f,2

=

ρ

2

ρ

sol

m

2

m

f,2

,

Y

f,i

=

m

2

m

f,i

+ 0.6

hR

aver

i,

(1)

Y

f

= ϕ

f,2

Y

f,2

+

X

i

ϕ

f,i

Y

f,i

,

i = H

2

, CH

4

, CO;

m

2

= ϕ

2

ρ

2

k

0,2

(ρ

s

C

s

) exp

−

E

s

RT

s

,

(2)

m

f,2

= k

0,2

(ρ

2

C

2

) (ρ

ox

C

ox

)exp

−

E

s

RT

f,2

,

(3)

m

f,i

= k

0,f,i

(ρC)

i

(ρ

ox

C

ox

)

ν

f,i

exp

−

E

f,i

RT

f,i

,

(4)

T

f,i

=

Q

f,i

c

p,g

+

T

heat

+ T

0

sol

2

.

(5)

Here ρ is the density, T is the temperature, m

2

and m

f

are the mass rates of the heterogeneous and gas-phase
reactions,

hR

aver

i is the statistical average pore radius,

ϕ is the volumetric fraction, E, ν, k

0

, and Q are the

activation energy, order, preexponent, and heat effect
of the chemical reaction, respectively, C is the mass
concentration, R is the universal gas constant, and c

p

is

the specific heat; the subscript “sol” refers to the solid,
2 to the soot-formation products, “f” to the flame, “s”
to the surface, “g” to the gas, “ox” to the oxidizer in the
external flow, “heat” to preheating, and the superscript
zero refers to the initial state.

The dimensionless heights of individual flames have

the form

ξ

f,2

=

c

p,g

λ

g

ρ

2

ρ

sol

m

2

Y

f,2

,

ξ

f,i

=

c

p,g

λ

g

m

2

Y

f,i

,

(6)

where λ

g

is the thermal conductivity of the gas.

Considering (1)–(6), we write the integral heat ef-

fect due to the gas-phase reactions in the flame:

Q

f,s

= ϕ

f,2

Q

s

Y

f,2

ρ

2

y

∗

ρ

sol

exp (

−ξ

f,2

)

+

X

i

ϕ

f,i

Q

f,i

ρ

f,i

y

∗

ρ

sol

Y

f,i

exp (

−ξ

f,i

).

(7)

Here y

∗

is the characteristic dimension of the chemical-

reaction zone. In this approach, the rate of change of
the reacting gas-phase components per unit solid surface
can be written as

ρ

f,s

Y

f

∂(ϕ

f

η

f,s

)

∂t

= ϕ

f,2

ρ

ox

C

ox

(Y

f,2

)

−1

ρ

2

k

0,2

× (1 − η

s,2

) exp

−

E

s

RT

s

+

X

i

ϕ

f,i

ρ

f,s

(Y

f,i

)

× (1 − η

s,i

) k

0,f,i

(ρ

ox

C

ox

)

ν

f,i

exp

−

E

f,i

RT

s

.

(8)

Thus, expressions (1)–(8) can be used to approxi-

mately describe the heat release due to gas-phase reac-
tions taking into account experimental information on
the quasistationarity of the gas-phase processes [4, 5]
during ignition of variously shaped specimens of CPM
by the mechanism described above.

An important problem of ignition theory for hetero-

geneous systems [5, 13] is the estimation of critical con-
ditions using the equality of heat input and heat removal
on the interface [13]. For the problem considered, the
critical condition is propagation of the heterogeneous
oxidation of soot-formation products at t > t

heat

into

the intradiffusion region [13]. In this case, the curves
of heat input and heat removal on the porous surface
must have a tangency point at a certain temperature
T

∗

. Then, taking into account (2) and the results of

[5, 13], we have

Parametric Analysis of the Ignition Conditions for Composite Polymeric Materials

557

p

Θ

heat

2ϕ

2

hR

aver

i/y

∗

q

2,s

k

0,2

(ρ

∗

C

ox

) exp

−

E

s

RT

∗

> σε

s

(T

4

∗

− T

4

ox

) + α

c

(T

∗

− T

ox

),

(9)

where Θ

heat

= E

s

(T

heat

−T

∗

)/RT

2

∗

is the dimensionless

surface temperature at t = t

heat

, α

c

is the convective

heat-transfer coefficient, ε

s

is the emissivity factor of

the surface, σ = 5.67

· 10

−8

W/(m

2

· K

4

) is the Stefan–

Boltzmann constant, and q

2,s

is the heat effect of the

heterogeneous reaction.

For convenience, the heat transfer due to reradia-

tion in expression (9) is written as follows [5]:

σε

s

(T

4

∗

− T

4

ox

) = σε

s

T

3

∗

h

1 +

T

ox

T

∗

+

T

ox

T

∗

2

+

T

ox

T

∗

3

i

(T

∗

− T

ox

). (10)

We denote the effective coefficient of heat transfer

due to reradiation at T

ox

T

∗

by

α

rad

= σε

s

T

3

∗

h

1 +

T

ox

T

∗

+

T

ox

T

∗

2

+

T

ox

T

∗

3

i

.

Then, on the right side of the balance relation (9),

we have the heat transfer to the cold oxidizer flow at
α = α

c

+α

rad

taking into account (10). In dimensionless

form, (9) is written as

p

Θ

heat

B

layer

∗

δ

s

> −Θ

ox

,

(11)

where

δ

s

=

E

s

q

2,s

k

0,2

(ρ

∗

C

ox

)

αRT

2

∗

exp

−

E

s

RT

∗

is the Frank-Kamenetskii parameter [13], B

layer

∗

=

2ϕ

2

y

∗

/

hR

aver

i is a parameter that describes the struc-

ture of the porous layer, and Θ

ox

= (T

ox

− T

∗

)E

s

/(RT

2

∗

)

is the dimensionless temperature of the external flow of
the oxidizer.

As the characteristic dimension y

∗

, it is necessary

to use the depth of penetration of the oxidation reaction
of the soot-formation products into the porous layer:

y

∗

=

pD

layer,ox

t

ign

.

(12)

Here t

ign

is the time of diffusion ignition reckoned from

t = t

heat

to the point of inflection on the curve of T

s

(t),

D

layer,ox

= D

ox

Π

2
layer

is the effective coefficient of dif-

fusion of the oxidizer in the porous layer [11, 14], D

ox

is the coefficient of binary diffusion, and Π

layer

is the

porosity of the layer.

As an example, we calculate the ignition conditions

for curve 3 in Fig. 5, using data from [4, 5, 10, 11, 13, 14].
Omitting intermediate evaluations of the quantities in-
cluded in relations (10)–(12), we obtain the following

values for the dimensionless parameters: Θ

heat

= 1.210,

B

layer

∗

= 22.656, δ

s

= 0.585, and Θ

ox

=

−13.287.

As follows from Fig. 5, diffusion ignition of soot-

formation products in the porous layer (see curve 3)
is observed with subsequent transition to self-sustained
combustion of a tubular CPM-2 specimen in the gaseous
oxidizer flow at C

ox

= 0.98. For this example, the classi-

cal Frank-Kamenetskii’s estimates [13] of heterogeneous
ignition conditions (limiting value δ

s

≈ e

−1

≈ 0.368) are

also valid. Indeed, if we decrease the oxygen concentra-
tion in the gas flow to the value C

ox

= 0.23 (the air), we

obtain δ

s

= 0.124. According to [13], the heterogeneous

ignition conditions do not occur, and for the data of
Fig. 5, the tubular specimens extinguishes, which was
confirmed experimentally (see the dashed curves).

In conclusion, it should be noted that using re-

lations (9) and (11), one can determine the unknown
characteristics of the porous layer in CPM (param-
eter B

layer

∗

) for specified macroscopic parameters of

the heterogeneous oxidation of the soot-formation prod-
ucts and convective heat-transfer coefficients or find un-
known macroscopic parameters of the reaction from the
known parameter B

layer

∗

.

Corresponding procedures have been elaborated for

different types of reactions (see, for example, the “rec-
tification” method in [5, 9, 11, 13, 14]).

CONCLUSIONS

1.

An experimental procedure is developed to

study the thermal destruction and ignition of hemi-
spherical, plate, and tubular specimen of CPM in gas
flows with variable properties.

2. Experiments showed the existence of three ig-

nition regimes of a preheated CPM specimen in a cold
gaseous oxidizer flow, one of which (extinction) indi-
cates the existence of critical conditions.

3. Probe thermocouple measurements of nonsta-

tionary temperature profiles in the gas and condensed
phases proved that at the moment of contact with the
oxidizer from the external flow, the gas-phase reactions
of the thermal destruction products of CPM are quasis-
tationary and their intensity does not have a predomi-
nant effect on the observed ignition regimes. Formulas
are given for calculation of the integral heat release from
these reactions on the surface using the model of com-
petitive flames.

4. The balance relation between the heat input and

heat removal on the porous reacting surface is formu-
lated, which allows one to estimate the critical condi-
tions of diffusion ignition of CPM under the examined
conditions.

It is interesting that this relation agrees

558

Isakov

with the classical concepts of heterogeneous ignition if
the parameter B

layer

∗

characterizing the diffusion mech-

anism of transfer of the oxidizer in the pores is taken
into account. This is confirmed by particular calcula-
tions and experiments.

REFERENCES

1. V. I. Al’perin, N. V. Korol’kov, A. V. Motavkin, et

al., Structural Fiberglass Plastics [in Russian], Khimiya,

Moscow (1979).

2. A. P. Shevchuk, O. A. Simonov, Yu. N. Shebeko, et

al., “Regularities of accidents with tanks with liquefied

hydrocarbon gases accompanied by formation of ‘fire

balls,’ ” Khim. Prom., No. 6, 18–20 (1991).

3. Yu. N. Shebeko, A. P. Shevchuk, I. M. Smolin, et al.,

“Fire and explosion safety in transportation of lique-

fied hydrocarbon of gases by rail. 2. Calculation of the

damaging factors of fire and explosion during depressur-

ization of a tank car with liquefied hydrocarbon gases,”

Pozharovzryvobezopasnost’, No. 1, 39–45 (1993).

4. G. N. Isakov and G. Ya. Mamontov, “Unsteady heat ex-

change and ignition of fiberglass plastics under variable

thermal loading,” in: Contribution of Young Scientists

and Specialists to National Economy, Proc. Regional

Sci. Conf., Izd. Tomsk. Univ., Tomsk (1977), pp. 203–

205.

5. G. N. Isakov, Heat and Mass Transfer and Ignition in

Heterogeneous Systems [in Russian], Izd. Sib. Otd. Ross.

Akad. Nauk, Novosibirsk (1999).

6. ISO 4589:1984. Plastics. Determination of Flammability

by Oxygen Index.

7. ISO 9773:1990. Plastics. Determination of Burning Be-

havior of Flexible Vertical Specimens in Contact with a

Small-Flame Ignition Source.

8. I. S. Reshetnikov, A. V. Antonov, and N. A. Khal-

turinskii, “Mathematical description of combustion of

intumescent polymer systems,” Combust. Expl. Shock

Waves, 33, No. 6, 669–684 (1997).

9. V. V. Nesmelov, G. N. Isakov, et al., “New data on

the regularities of thermal destruction of polymeric com-

pounds under convective heat and mass transfer,” Dokl.

Akad. Nauk SSSR, 292, No. 5, 1123–1126 (1987).

10. V. V. Nesmelov, “Effect of preliminary thermal treat-

ment on the ignitability of coking polymers,” Tomsk

(1987). Deposited at VINITI 06.05.87, No. 4047–B87.

11. N. V. Lavrov, Physicochemical Fundamentals of Fuel

Combustion [in Russian], Nauka, Moscow (1971).

12. E. S. Shchetinkov, Physics of Gas Combustion [in Rus-

sian], Nauka, Moscow (1965).

13. D. A. Frank-Kamenetskii, Diffusion and Heat Trans-

fer in Chemical Kinetics [in Russian], Nauka, Moscow

(1987).

14. M. S. Orenbakh, Reaction Surface in Heterogeneous

Combustion [in Russian], Nauka, Novosibirsk (1973).